4–2C Is the boundary work associated with constant-volume systems always zero? Get 4.2 exercise solution
4–3C An ideal gas at a given state expands to a fixed final volume first at constant pressure and then at constant temperature. For which case is the work done greater? Get 4.3 exercise solution
4–4C Show that 1 kPa · m3 1 kJ. Get 4.4 exercise solution
4–5 A piston–cylinder device initially contains 0.07 m3 of nitrogen gas at 130 kPa and 120°C. The nitrogen is now expanded polytropically to a state of 100 kPa and 100°C. Determine the boundary work done during this process. Get 4.5 exercise solution
4–6 A piston–cylinder device with a set of stops initially contains 0.3 kg of steam at 1.0 MPa and 400°C. The location of the stops corresponds to 60 percent of the initial volume. Now the steam is cooled. Determine the compression work if the final state is (a) 1.0 MPa and 250°C and (b) 500 kPa. (c) Also determine the temperature at the final state in part (b) Get 4.6 exercise solution
4–7 A piston–cylinder device initially contains 0.07 m3 of nitrogen gas at 130 kPa and 120°C. The nitrogen is now expanded to a pressure of 100 kPa polytropically with a polytropic exponent whose value is equal to the specific heat ratio (called isentropic expansion). Determine the final temperature and the boundary work done during this process. Get 4.7 exercise solution
4–8 A mass of 5 kg of saturated water vapor at 300 kPa is heated at constant pressure until the temperature reaches 200°C. Calculate the work done by the steam during this process. Get 4.8 exercise solution
4–9 A frictionless piston–cylinder device initially contains 200 L of saturated liquid refrigerant-134a. The piston is free to move, and its mass is such that it maintains a pressure of 900 kPa on the refrigerant. The refrigerant is now heated until its temperature rises to 70°C. Calculate the work done during this process. Get 4.9 exercise solution
4–10 Reconsider Prob. 4–9. Using EES (or other) software, investigate the effect of pressure on the work done. Let the pressure vary from 400 kPa to 1200 kPa. Plot the work done versus the pressure, and discuss the results. Explain why the plot is not linear. Also plot the process described in Prob. 4–9 on the P-v diagram. Get 4.10 exercise solution
4–11E A frictionless piston–cylinder device contains 16 lbm of superheated water vapor at 40 psia and 600°F. Steam is now cooled at constant pressure until 70 percent of it, by mass, condenses. Determine the work done during this process. Get 4.11 exercise solution
4–12 A mass of 2.4 kg of air at 150 kPa and 12°C is contained in a gas-tight, frictionless piston–cylinder device. The air is now compressed to a final pressure of 600 kPa. During the process, heat is transferred from the air such that the temperature inside the cylinder remains constant. Calculate the work input during this process. Get 4.12 exercise solution
4–13 Nitrogen at an initial state of 300 K, 150 kPa, and 0.2 m3 is compressed slowly in an isothermal process to a final pressure of 800 kPa. Determine the work done during this process. Get 4.13 exercise solution
4–14 A gas is compressed from an initial volume of 0.42 m3 to a final volume of 0.12 m3. During the quasi-equilibrium Get 4.14 exercise solution
4–15E During an expansion process, the pressure of a gas changes from 15 to 100 psia according to the relation P = aV + b, where a = 5 psia/ft3 and b is a constant. If the initial volume of the gas is 7 ft3, calculate the work done during the process. Get 4.15 exercise solution
4–16 During some actual expansion and compression processes in piston–cylinder devices, the gases have been observed to satisfy the relationship PVn = C, where n and C are constants. Calculate the work done when a gas expands from 150 kPa and 0.03 m3 to a final volume of 0.2 m3 for the case of n = 1.3. Get 4.16 exercise solution
4–17 Reconsider Prob. 4–16. Using the EES (or other) software, plot the process described in the problem on a P-V diagram, and investigate the effect of the polytropic exponent n on the boundary work. Let the polytropic exponent vary from 1.1 to 1.6. Plot the boundary work versus the polytropic exponent, and discuss the results. Get 4.17 exercise solution
4–18 A frictionless piston–cylinder device contains 2 kg of nitrogen at 100 kPa and 300 K. Nitrogen is now compressed slowly according to the relation PV1.4 constant until it reaches a final temperature of 360 K. Calculate the work input during this process. Get 4.18 exercise solution
4–19 The equation of state of a gas is given as (P = 10/ 2) = RuT, where the units of and P are m3/kmol and kPa, respectively. Now 0.5 kmol of this gas is expanded in a quasi-equilibrium manner from 2 to 4 m3 at a constant temperature of 300 K. Determine (a) the unit of the quantity 10 in the equation and (b) the work done during this isothermal expansion process. Get 4.19 exercise solution
4–20 Reconsider Prob. 4–19. Using the integration feature of the EES software, calculate the work done, and compare your result with the “hand-calculated” result obtained in Prob. 4–19. Plot the process described in the problem on a P-v diagram. Get 4.20 exercise solution
4–21 Carbon dioxide contained in a piston–cylinder device is compressed from 0.3 to 0.1 m3. During the process, the pressure and volume are related by P = aV-2, where a = 8 kPa · m6. Calculate the work done on the carbon dioxide during this process. Get 4.21 exercise solution
4–22E Hydrogen is contained in a piston–cylinder device at 14.7 psia and 15 ft3. At this state, a linear spring (F ∝ x) with a spring constant of 15,000 lbf/ft is touching the piston but exerts no force on it. The cross-sectional area of the piston is 3 ft2. Heat is transferred to the hydrogen, causing it to expand until its volume doubles. Determine (a) the final pressure, (b) the total work done by the hydrogen, and (c) the fraction of this work done against the spring. Also, show the process on a P-V diagram. Get 4.22 exercise solution
4–23 A piston–cylinder device contains 50 kg of water at 250 kPa and 25°C. The cross-sectional area of the piston is 0.1 m2. Heat is now transferred to the water, causing part of it to evaporate and expand. When the volume reaches 0.2 m3, the piston reaches a linear spring whose spring constant is 100 kN/m. More heat is transferred to the water until the piston rises 20 cm more. Determine (a) the final pressure and temperature and (b) the work done during this process. Also, show the process on a P-V diagram. Get 4.23 exercise solution
4–24 Reconsider Prob. 4–23. Using the EES software, investigate the effect of the spring constant on the final pressure in the cylinder and the boundary work done. Let the spring constant vary from 50 kN/m to 500 kN/m. Plot the final pressure and the boundary work against the spring constant, and discuss the results. Get 4.24 exercise solution
4–25 Determine the boundary work done by a gas during an expansion process if the pressure and volume values at various states are measured to be 300 kPa, 1 L; 290 kPa, 1.1 L; 270 kPa, 1.2 L; 250 kPa, 1.4 L; 220 kPa, 1.7 L; and 200 kPa, 2 L. Get 4.25 exercise solution
4–26 A piston–cylinder device initially contains 0.25 kg of nitrogen gas at 130 kPa and 120°C. The nitrogen is now expanded isothermally to a pressure of 100 kPa. Determine the boundary work done during this process. Get 4.26 exercise solution
4–27 A piston–cylinder device contains 0.15 kg of air initially at 2 MPa and 350°C. The air is first expanded isothermally to 500 kPa, then compressed polytropically with a polytropic exponent of 1.2 to the initial pressure, and finally compressed at the constant pressure to the initial state. Determine the boundary work for each process and the net work of the cycle. Get 4.27 exercise solution
4–28 A 0.5-m3 rigid tank contains refrigerant-134a initially at 160 kPa and 40 percent quality. Heat is now transferred to the refrigerant until the pressure reaches 700 kPa. Determine (a) the mass of the refrigerant in the tank and (b) the amount of heat transferred. Also, show the process on a P-v diagram with respect to saturation lines. Get 4.28 exercise solution
4–29E A 20-ft3 rigid tank initially contains saturated refrigerant-134a vapor at 160 psia. As a result of heat transfer from the refrigerant, the pressure drops to 50 psia. Show the process on a P-v diagram with respect to saturation lines, and determine (a) the final temperature, (b) the amount of refrigerant that has condensed, and (c) the heat transfer. Get 4.29 exercise solution
4–30 A well-insulated rigid tank contains 5 kg of a saturated liquid–vapor mixture of water at l00 kPa. Initially, three-quarters of the mass is in the liquid phase. An electric resistor placed in the tank is connected to a 110-V source, and a current of 8 A flows through the resistor when the switch is turned on. Determine how long it will take to vaporize all the liquid in the tank. Also, show the process on a T-v diagram with respect to saturation lines.

Get 4.30 exercise solution
4–31 Reconsider Prob. 4–30. Using EES (or other) software, investigate the effect of the initial mass of water on the length of time required to completely vaporize the liquid. Let the initial mass vary from 1 to 10 kg. Plot the vaporization time against the initial mass, and discuss the results. Get 4.31 exercise solution
4–32 An insulated tank is divided into two parts by a partition. One part of the tank contains 2.5 kg of compressed liquid water at 60°C and 600 kPa while the other part is evacuated. The partition is now removed, and the water expands to fill the entire tank. Determine the final temperature of the water and the volume of the tank for a final pressure of 10 kPa. Get 4.32 exercise solution
4–33 Reconsider Prob. 4–32. Using EES (or other) software, investigate the effect of the initial pressure of water on the final temperature in the tank. Let the initial pressure vary from 100 to 600 kPa. Plot the final temperature against the initial pressure, and discuss the results. Get 4.33 exercise solution
4–34 A piston–cylinder device contains 5 kg of refrigerant134a at 800 kPa and 70°C. The refrigerant is now cooled at constant pressure until it exists as a liquid at 15°C. Determine the amount of heat loss and show the process on a T-v diagram with respect to saturation lines. Get 4.34 exercise solution
4–35E A piston–cylinder device contains 0.5 lbm of water initially at 120 psia and 2 ft3. Now 200 Btu of heat is transferred to the water while its pressure is held constant. Determine the final temperature of the water. Also, show the process on a T-v diagram with respect to saturation lines. Get 4.35 exercise solution
4–36 An insulated piston–cylinder device contains 5 L of saturated liquid water at a constant pressure of 175 kPa. Water is stirred by a paddle wheel while a current of 8 A flows for 45 min through a resistor placed in the water. If one-half of the liquid is evaporated during this constantpressure process and the paddle-wheel work amounts to 400 kJ, determine the voltage of the source. Also, show the process on a P-v diagram with respect to saturation lines.

Get 4.36 exercise solution
4–37 A piston–cylinder device contains steam initially at 1 MPa, 450°C, and 2.5 m3. Steam is allowed to cool at constant pressure until it first starts condensing. Show the process on a T-v diagram with respect to saturation lines and determine (a) the mass of the steam, (b) the final temperature, and (c) the amount of heat transfer. Get 4.37 exercise solution
4–38 A piston–cylinder device initially contains steam at 200 kPa, 200°C, and 0.5 m3. At this state, a linear spring (F x) is touching the piston but exerts no force on it. Heat is now slowly transferred to the steam, causing the pressure and the volume to rise to 500 kPa and 0.6 m3, respectively. Show the process on a P-v diagram with respect to saturation lines and determine (a) the final temperature, (b) the work done by the steam, and (c) the total heat transferred. Get 4.38 exercise solution
4–39 Reconsider Prob. 4–38. Using EES (or other) software, investigate the effect of the initial temperature of steam on the final temperature, the work done, and the total heat transfer. Let the initial temperature vary from 150 to 250°C. Plot the final results against the initial temperature, and discuss the results. Get 4.39 exercise solution
4–40 A piston–cylinder device initially contains 0.8 m3 of saturated water vapor at 250 kPa. At this state, the piston is resting on a set of stops, and the mass of the piston is such that a pressure of 300 kPa is required to move it. Heat is now slowly transferred to the steam until the volume doubles. Show the process on a P-v diagram with respect to saturation lines and determine (a) the final temperature, (b) the work done during this process, and (c) the total heat transfer Get 4.40 exercise solution
4–41 Two tanks (Tank A and Tank B) are separated by a partition. Initially Tank A contains 2-kg steam at 1 MPa and 300°C while Tank B contains 3-kg saturated liquid–vapor mixture with a vapor mass fraction of 50 percent. Now the partition is removed and the two sides are allowed to mix until the mechanical and thermal equilibrium are established. If the pressure at the final state is 300 kPa, determine (a) the temperature and quality of the steam (if mixture) at the final state and (b) the amount of heat lost from the tanks.

Get 4.41 exercise solution
4–42 A 30-L electrical radiator containing heating oil is placed in a 50-m3 room. Both the room and the oil in the radiator are initially at 10°C. The radiator with a rating of 1.8 kW is now turned on. At the same time, heat is lost from the room at an average rate of 0.35 kJ/s. After some time, the average temperature is measured to be 20°C for the air in the room, and 50°C for the oil in the radiator. Taking the density and the specific heat of the oil to be 950 kg/m3 and 2.2 kJ/kg . °C, respectively, determine how long the heater is kept on. Assume the room is well-sealed so that there are no air leaks. Get 4.42 exercise solution
4–43C Is the relation Du = mcv,avgDT restricted to constantvolume processes only, or can it be used for any kind of process of an ideal gas? Get 4.43 exercise solution
4–44C Is the relation Dh = mcp,avgDT restricted to constantpressure processes only, or can it be used for any kind of process of an ideal gas? Get 4.44 exercise solution
4–45C Show that for an ideal gas cp = cv + Ru. Get 4.45 exercise solution
4–46C Is the energy required to heat air from 295 to 305 K the same as the energy required to heat it from 345 to 355 K? Assume the pressure remains constant in both cases. Get 4.46 exercise solution
4–47C In the relation Du = mcv DT, what is the correct unit of cv — kJ/kg · °C or kJ/kg · K? Get 4.47 exercise solution
4–48C A fixed mass of an ideal gas is heated from 50 to 80°C at a constant pressure of (a) 1 atm and (b) 3 atm. For which case do you think the energy required will be greater? Why? Get 4.48 exercise solution
4–49C A fixed mass of an ideal gas is heated from 50 to 80°C at a constant volume of (a) 1 m3 and (b) 3 m3. For which case do you think the energy required will be greater? Why? Get 4.49 exercise solution
4–50C A fixed mass of an ideal gas is heated from 50 to 80°C (a) at constant volume and (b) at constant pressure. For which case do you think the energy required will be greater? Why? Get 4.50 exercise solution
4–51 Determine the enthalpy change Dh of nitrogen, in kJ/kg, as it is heated from 600 to 1000 K, using (a) the empirical specific heat equation as a function of temperature (Table A–2c), (b) the cp value at the average temperature(Table A–2b), and (c) the cp value at room temperature (Table A–2a). Get 4.51 exercise solution
4–52E Determine the enthalpy change Dh of oxygen, in Btu/lbm, as it is heated from 800 to 1500 R, using (a) the empirical specific heat equation as a function of temperature (Table A–2Ec), (b) the cp value at the average temperature (Table A–2Eb), and (c) the cp value at room temperature (Table A–2Ea). Get 4.52 exercise solution
4–53 Determine the internal energy change Du of hydrogen, in kJ/kg, as it is heated from 200 to 800 K, using (a) the empirical specific heat equation as a function of temperature (Table A–2c), (b) the cv value at the average temperature (Table A–2b), and (c) the cv value at room temperature (Table A–2a). Get 4.53 exercise solution
4–54C Is it possible to compress an ideal gas isothermally in an adiabatic piston–cylinder device? Explain. Get 4.54 exercise solution
4–55E A rigid tank contains 20 lbm of air at 50 psia and 80°F. The air is now heated until its pressure doubles. Determine (a) the volume of the tank and (b) the amount of heat transfer. Answers: (a) 80 ft3,( b) 1898 Btu Get 4.55 exercise solution
4–56 A 3-m3 rigid tank contains hydrogen at 250 kPa and 550 K. The gas is now cooled until its temperature drops to 350 K. Determine (a) the final pressure in the tank and (b) the amount of heat transfer. Get 4.56 exercise solution
4–57 A 4-m x 5-m x 6-m room is to be heated by a baseboard resistance heater. It is desired that the resistance heater be able to raise the air temperature in the room from 7 to 23°C within 15 min. Assuming no heat losses from the room and an atmospheric pressure of 100 kPa, determine the required power of the resistance heater. Assume constant specific heats at room temperature. Get 4.57 exercise solution
4–58 A 4-m x 5-m x 7-m room is heated by the radiator of a steam-heating system. The steam radiator transfers heat at a rate of 10,000 kJ/h, and a 100-W fan is used to distribute the warm air in the room. The rate of heat loss from the room is estimated to be about 5000 kJ/h. If the initial temperature of the room air is 10°C, determine how long it will take for the air temperature to rise to 20°C. Assume constant specific heats at room temperature.
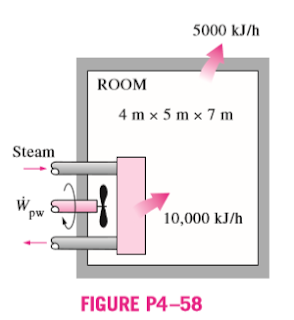
Get 4.58 exercise solution
4–59 A student living in a 4-m x 6-m x 6-m dormitory room turns on her 150-W fan before she leaves the room on a summer day, hoping that the room will be cooler when she comes back in the evening. Assuming all the doors and windows are tightly closed and disregarding any heat transfer through the walls and the windows, determine the temperature in the room when she comes back 10 h later. Use specific heat values at room temperature, and assume the room to be at 100 kPa and 15°C in the morning when she leaves. Get 4.59 exercise solution
4–60E A 10-ft3 tank contains oxygen initially at 14.7 psia and 80°F. A paddle wheel within the tank is rotated until the pressure inside rises to 20 psia. During the process 20 Btu of heat is lost to the surroundings. Determine the paddle-wheel work done. Neglect the energy stored in the paddle wheel. Get 4.60 exercise solution
4–61 An insulated rigid tank is divided into two equal parts by a partition. Initially, one part contains 4 kg of an ideal gas at 800 kPa and 50°C, and the other part is evacuated. The partition is now removed, and the gas expands into the entire tank. Determine the final temperature and pressure in the tank Get 4.61 exercise solution
4–62 A piston–cylinder device whose piston is resting on top of a set of stops initially contains 0.5 kg of helium gas at 100 kPa and 25°C. The mass of the piston is such that 500 kPa of pressure is required to raise it. How much heat must be transferred to the helium before the piston starts rising? Get 4.62 exercise solution
4–63 An insulated piston–cylinder device contains 100 L of air at 400 kPa and 25°C. A paddle wheel within the cylinder is rotated until 15 kJ of work is done on the air while the pressure is held constant. Determine the final temperature of the air. Neglect the energy stored in the paddle wheel. Get 4.63 exercise solution
4–64E A piston–cylinder device contains 25 ft3 of nitrogen at 40 psia and 700°F. Nitrogen is now allowed to cool at constant pressure until the temperature drops to 200°F. Using specific heats at the average temperature, determine the amount of heat loss. Get 4.64 exercise solution
4–65 A mass of 15 kg of air in a piston–cylinder device is heated from 25 to 77°C by passing current through a resistance heater inside the cylinder. The pressure inside the cylinder is held constant at 300 kPa during the process, and a heat loss of 60 kJ occurs. Determine the electric energy supplied, in kWh

Get 4.65 exercise solution
4–66 An insulated piston–cylinder device initially contains 0.3 m3 of carbon dioxide at 200 kPa and 27°C. An electric switch is turned on, and a 110-V source supplies current to a resistance heater inside the cylinder for a period of 10 min. The pressure is held constant during the process, while the volume is doubled. Determine the current that passes through the resistance heater. Get 4.66 exercise solution
4–67 A piston–cylinder device contains 0.8 kg of nitrogen initially at 100 kPa and 27°C. The nitrogen is now compressed slowly in a polytropic process during which PV1.3 constant until the volume is reduced by one-half. Determine the work done and the heat transfer for this process. Get 4.67 exercise solution
4–68 Reconsider Prob. 4–67. Using EES (or other) software, plot the process described in the problem on a P-V diagram, and investigate the effect of the polytropic exponent n on the boundary work and heat transfer. Let the polytropic exponent vary from 1.1 to 1.6. Plot the boundary work and the heat transfer versus the polytropic exponent, and discuss the results. Get 4.68 exercise solution
4–69 A room is heated by a baseboard resistance heater. When the heat losses from the room on a winter day amount to 6500 kJ/h, the air temperature in the room remains constant even though the heater operates continuously. Determine the power rating of the heater, in kW.

Get 4.69 exercise solution
4–70E A piston–cylinder device contains 3 ft3 of air at 60 psia and 150°F. Heat is transferred to the air in the amount of 40 Btu as the air expands isothermally. Determine the amount of boundary work done during this process. Get 4.70 exercise solution
4–71 A piston–cylinder device contains 4 kg of argon at 250 kPa and 35°C. During a quasi-equilibrium, isothermal expansion process, 15 kJ of boundary work is done by the system, and 3 kJ of paddle-wheel work is done on the system. Determine the heat transfer for this process. Get 4.71 exercise solution
4–72 A piston–cylinder device, whose piston is resting on a set of stops, initially contains 3 kg of air at 200 kPa and 27°C. The mass of the piston is such that a pressure of 400 kPa is required to move it. Heat is now transferred to the air until its volume doubles. Determine the work done by the air and the total heat transferred to the air during this process. Also show the process on a P-v diagram. Get 4.72 exercise solution
4–73 A piston–cylinder device, with a set of stops on the top, initially contains 3 kg of air at 200 kPa and 27°C. Heat is now transferred to the air, and the piston rises until it hits the stops, at which point the volume is twice the initial volume. More heat is transferred until the pressure inside the cylinder also doubles. Determine the work done and the amount of heat transfer for this process. Also, show the process on a P-v diagram. Get 4.73 exercise solution
4–74 In a manufacturing facility, 5-cm-diameter brass balls (r = 8522 kg/m3 and cp = 0.385 kJ/kg · °C) initially at 120°C are quenched in a water bath at 50°C for a period of 2 min at a rate of 100 balls per minute. If the temperature of the balls after quenching is 74°C, determine the rate at which heat needs to be removed from the water in order to keep its temperature constant at 50°C. Get 4.74 exercise solution
4–75 Repeat Prob. 4–74 for aluminum balls. Get 4.75 exercise solution
4–76E During a picnic on a hot summer day, all the cold drinks disappeared quickly, and the only available drinks were those at the ambient temperature of 75°F. In an effort to cool a 12-fluid-oz drink in a can, a person grabs the can and starts shaking it in the iced water of the chest at 32°F. Using the properties of water for the drink, determine the mass of ice that will melt by the time the canned drink cools to 45°F. Get 4.76 exercise solution
4–77 Consider a 1000-W iron whose base plate is made of 0.5-cm-thick aluminum alloy 2024-T6 (r = 2770 kg/m3 and cp = 875 J/kg · °C). The base plate has a surface area of 0.03 m2. Initially, the iron is in thermal equilibrium with the ambient air at 22°C. Assuming 85 percent of the heat generated in the resistance wires is transferred to the plate, determine the minimum time needed for the plate temperature to reach 140°C. Get 4.77 exercise solution
4–78 Stainless steel ball bearings (r = 8085 kg/m3 and cp = 0.480 kJ/kg · °C) having a diameter of 1.2 cm are to be quenched in water at a rate of 800 per minute. The balls leave the oven at a uniform temperature of 900°C and are exposed to air at 25°C for a while before they are dropped into the water. If the temperature of the balls drops to 850°C prior to quenching, determine the rate of heat transfer from the balls to the air. Get 4.78 exercise solution
4–79 Carbon steel balls (r = 7833 kg/m3 and cp = 0.465 kJ/kg · °C) 8 mm in diameter are annealed by heating them first to 900°C in a furnace, and then allowing them to cool slowly to 100°C in ambient air at 35°C. If 2500 balls are to be annealed per hour, determine the total rate of heat transfer from the balls to the ambient air. Get 4.79 exercise solution
4–80 An electronic device dissipating 30 W has a mass of 20 g and a specific heat of 850 J/kg · °C. The device is lightly used, and it is on for 5 min and then off for several hours, during which it cools to the ambient temperature of 25°C. Determine the highest possible temperature of the device at the end of the 5-min operating period. What would your answer be if the device were attached to a 0.2-kg aluminum heat sink? Assume the device and the heat sink to be nearly isothermal. Get 4.80 exercise solution
4–81 Reconsider Prob. 4–80. Using EES (or other) software, investigate the effect of the mass of the heat sink on the maximum device temperature. Let the mass of heat sink vary from 0 to 1 kg. Plot the maximum temperature against the mass of heat sink, and discuss the results. Get 4.81 exercise solution
4–82 An ordinary egg can be approximated as a 5.5-cmdiameter sphere. The egg is initially at a uniform temperature of 8°C and is dropped into boiling water at 97°C. Taking the properties of the egg to be r = 1020 kg/m3 and cp = 3.32 kJ/kg · °C, determine how much heat is transferred to the egg by the time the average temperature of the egg rises to 80°C. Get 4.82 exercise solution
4–83E ln a production facility, 1.2-in-thick 2-ft = 2-ft square brass plates (r = 532.5 lbm/ft3 and cp = 0.091 Btu/lbm · °F) that are initially at a uniform temperature of 75°F are heated by passing them through an oven at 1300°F at a rate of 300 per minute. If the plates remain in the oven until their average temperature rises to 1000°F, determine the rate of heat transfer to the plates in the furnace. Get 4.83 exercise solution
4–84 Long cylindrical steel rods (r = 7833 kg/m3 and cp = 0.465 kJ/kg · °C) of 10-cm diameter are heat-treated by drawing them at a velocity of 3 m/min through an oven maintained at 900°C. If the rods enter the oven at 30°C and leave at a mean temperature of 700°C, determine the rate of heat transfer to the rods in the oven. Get 4.84 exercise solution
4–85C What is metabolism? What is basal metabolic rate? What is the value of basal metabolic rate for an average man? Get 4.85 exercise solution
4–86C For what is the energy released during metabolism in humans used? Get 4.86 exercise solution
4–87C Is the metabolizable energy content of a food the same as the energy released when it is burned in a bomb calorimeter? If not, how does it differ? Get 4.87 exercise solution
4–88C Is the number of prospective occupants an important consideration in the design of heating and cooling systems of classrooms? Explain. Get 4.88 exercise solution
4–89C What do you think of a diet program that allows for generous amounts of bread and rice provided that no butter or margarine is added? Get 4.89 exercise solution
4–90 Consider two identical rooms, one with a 2-kW electric resistance heater and the other with three couples fast dancing. In which room will the air temperature rise faster? Get 4.90 exercise solution
4–91 Consider two identical 80-kg men who are eating identical meals and doing identical things except that one of them jogs for 30 min every day while the other watches TV. Determine the weight difference between the two in a month. Get 4.91 exercise solution
4–92 Consider a classroom that is losing heat to the outdoors at a rate of 20,000 kJ/h. If there are 30 students in class, each dissipating sensible heat at a rate of 100 W, determine if it is necessary to turn the heater in the classroom on to prevent the room temperature from dropping. Get 4.92 exercise solution
4–93 A 68-kg woman is planning to bicycle for an hour. If she is to meet her entire energy needs while bicycling by eating 30-g chocolate candy bars, determine how many candy bars she needs to take with her. Get 4.93 exercise solution
4–94 A 55-kg man gives in to temptation and eats an entire 1-L box of ice cream. How long does this man need to jog to burn off the calories he consumed from the ice cream? Answer: 2.52 h Get 4.94 exercise solution
4–95 Consider a man who has 20 kg of body fat when he goes on a hunger strike. Determine how long he can survive on his body fat alone. Get 4.95 exercise solution
4–96 Consider two identical 50-kg women, Candy and Wendy, who are doing identical things and eating identical food except that Candy eats her baked potato with four teaspoons of butter while Wendy eats hers plain every evening. Determine the difference in the weights of Candy and Wendy after one year. Get 4.96 exercise solution
4–97 A woman who used to drink about one liter of regular cola every day switches to diet cola (zero calorie) and starts eating two slices of apple pie every day. Is she now consuming fewer or more calories? Get 4.97 exercise solution
4–98 A 60-kg man used to have an apple every day after dinner without losing or gaining any weight. He now eats a 200-ml serving of ice cream instead of an apple and walks 20 min every day. On this new diet, how much weight will he lose or gain per month? Get 4.98 exercise solution
4–99 The average specific heat of the human body is 3.6 kJ/kg · °C. If the body temperature of an 80-kg man rises from 37°C to 39°C during strenuous exercise, determine the increase in the thermal energy of the body as a result of this rise in body temperature. Get 4.99 exercise solution
4–100E Alcohol provides 7 Calories per gram, but it provides no essential nutrients. A 1.5 ounce serving of 80-proof liquor contains 100 Calories in alcohol alone. Sweet wines and beer provide additional calories since they also contain carbohydrates. About 75 percent of American adults drink some sort of alcoholic beverage, which adds an average of 210 Calories a day to their diet. Determine how many pounds less an average American adult will weigh per year if he or she quit drinking alcoholic beverages and started drinking diet soda. Get 4.100 exercise solution
4–101 A 12-oz serving of a regular beer contains 13 g of alcohol and 13 g of carbohydrates, and thus 150 Calories. A 12-oz serving of a light beer contains 11 g of alcohol and 5 g of carbohydrates, and thus 100 Calories. An average person burns 700 Calories per hour while exercising on a treadmill. Determine how long it will take to burn the calories from a 12-oz can of (a) regular beer and (b) light beer on a treadmill. Get 4.101 exercise solution
4–102 A 5-oz serving of a Bloody Mary contains 14 g of alcohol and 5 g of carbohydrates, and thus 116 Calories. A 2.5-oz serving of a martini contains 22 g of alcohol and a negligible amount of carbohydrates, and thus 156 Calories. An average person burns 600 Calories per hour while exercising on a cross-country ski machine. Determine how long it will take to burn the calories from one serving of (a) a Bloody Mary and (b) a martini on this cross-country ski machine. Get 4.102 exercise solution
4–103E A 176-pound man and a 132-pound woman went to Burger King for lunch. The man had a BK Big Fish sandwich (720 Cal), medium french fries (400 Cal), and a large Coke (225 Cal). The woman had a basic hamburger (330 Cal), medium french fries (400 Cal), and a diet Coke (0 Cal). After lunch, they start shoveling snow and burn calories at a rate of 360 Cal/h for the woman and 480 Cal/h for the man. Determine how long each one of them needs to shovel snow to burn off the lunch calories. Get 4.103 exercise solution
4–104 Consider two friends who go to Burger King every day for lunch. One of them orders a Double Whopper sandwich, large fries, and a large Coke (total Calories = 1600) while the other orders a Whopper Junior, small fries, and a small Coke (total Calories = 800) every day. If these two friends are very much alike otherwise and they have the same metabolic rate, determine the weight difference between these two friends in a year. Get 4.104 exercise solution
4–105E A 150-pound person goes to Hardee’s for dinner and orders a regular roast beef (270 Cal) and a big roast beef (410 Cal) sandwich together with a 12-oz can of Pepsi (150 Cal). A 150-pound person burns 400 Calories per hour while climbing stairs. Determine how long this person needs to climb stairs to burn off the dinner calories. Get 4.105 exercise solution
4–106 A person eats a McDonald’s Big Mac sandwich (530 Cal), a second person eats a Burger King Whopper sandwich (640 Cal), and a third person eats 50 olives with regular french fries (350 Cal) for lunch. Determine who consumes the most calories. An olive contains about 5 Calories. Get 4.106 exercise solution
4–107 A 100-kg man decides to lose 5 kg without cutting down his intake of 3000 Calories a day. Instead, he starts fast swimming, fast dancing, jogging, and biking each for an hour every day. He sleeps or relaxes the rest of the day. Determine how long it will take him to lose 5 kg. Get 4.107 exercise solution
4–108E The range of healthy weight for adults is usually expressed in terms of the body mass index (BMI), defined, in SI units, as where W is the weight (actually, the mass) of the person in kg and H is the height in m, and the range of healthy weight is 19 <= BMI >= 25.
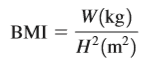
Convert the previous formula to English units such that the weight is in pounds and the height in inches. Also, calculate your own BMI, and if it is not in the healthy range, determine how many pounds (or kg) you need to gain or lose to be fit. Get 4.108 exercise solution
4–109 The body mass index (BMI) of a 1.7-m tall woman who normally has 3 large slices of cheese pizza and a 400-ml Coke for lunch is 30. She now decides to change her lunch to 2 slices of pizza and a 200-ml Coke. Assuming that the deficit in the calorie intake is made up by burning body fat, determine how long it will take for the BMI of this person to drop to 25. Use the data in the text for calories and take the metabolizable energy content of 1 kg of body fat to be 33,100 kJ. Get 4.109 exercise solution
4–110 Consider a piston–cylinder device that contains 0.5 kg air. Now, heat is transferred to the air at constant pressure and the air temperature increases by 5°C. Determine the expansion work done during this process. Get 4.110 exercise solution
4–111 In solar-heated buildings, energy is often stored as sensible heat in rocks, concrete, or water during the day for use at night. To minimize the storage space, it is desirable to use a material that can store a large amount of heat while experiencing a small temperature change. A large amount of heat can be stored essentially at constant temperature during a phase change process, and thus materials that change phase at about room temperature such as glaubers salt (sodium sulfate decahydrate), which has a melting point of 32°C and a heat of fusion of 329 kJ/L, are very suitable for this purpose. Determine how much heat can be stored in a 5-m3 storage space using (a) glaubers salt undergoing a phase change, (b) granite rocks with a heat capacity of 2.32 kJ/kg · °C and a temperature change of 20°C, and (c) water with a heat capacity of 4.00 kJ/kg · °C and a temperature change of 20°C. Get 4.111 exercise solution
4–112 A piston–cylinder device contains 0.8 kg of an ideal gas. Now, the gas is cooled at constant pressure until its temperature decreases by 10°C. If 16.6 kJ of compression work is done during this process, determine the gas constant and the molar mass of the gas. Also, determine the constantvolume and constant-pressure specific heats of the gas if its specific heat ratio is 1.667. Get 4.112 exercise solution
4–113 The temperature of air changes from 0 to 10°C while its velocity changes from zero to a final velocity, and its elevation changes from zero to a final elevation. At which values of final air velocity and final elevation will the internal, kinetic, and potential energy changes be equal? Answers: 119.8 m/s, 731.9 m Get 4.113 exercise solution
4–114 A frictionless piston–cylinder device initially contains air at 200 kPa and 0.2 m3. At this state, a linear spring (F ∝ x) is touching the piston but exerts no force on it. The air is now heated to a final state of 0.5 m3 and 800 kPa. Determine (a) the total work done by the air and (b) the work done against the spring. Also, show the process on a P-v diagram Get 4.114 exercise solution
4–115 A mass of 5 kg of saturated liquid–vapor mixture of water is contained in a piston–cylinder device at 125 kPa. Initially, 2 kg of the water is in the liquid phase and the rest is in the vapor phase. Heat is now transferred to the water, and the piston, which is resting on a set of stops, starts moving when the pressure inside reaches 300 kPa. Heat transfer continues until the total volume increases by 20 percent. Determine (a) the initial and final temperatures, (b) the mass of liquid water when the piston first starts moving, and (c) the work done during this process. Also, show the process on a P-v diagram. Get 4.115 exercise solution
4–116E A spherical balloon contains 10 lbm of air at 30 psia and 800 R. The balloon material is such that the pressure inside is always proportional to the square of the diameter. Determine the work done when the volume of the balloon doubles as a result of heat transfer. Answer: 715 Btu Get 4.116 exercise solution
4–117E Reconsider Prob. 4–116E. Using the integration feature of the EES software, determine the work done. Compare the result with your “handcalculated” result. Get 4.117 exercise solution
4–118 A mass of 12 kg of saturated refrigerant-134a vapor is contained in a piston–cylinder device at 240 kPa. Now 300 kJ of heat is transferred to the refrigerant at constant pressure while a 110-V source supplies current to a resistor within the cylinder for 6 min. Determine the current supplied if the final temperature is 70°C. Also, show the process on a T-v diagram with respect to the saturation lines. Get 4.118 exercise solution
4–119 A mass of 0.2 kg of saturated refrigerant-134a is contained in a piston–cylinder device at 200 kPa. Initially, 75 percent of the mass is in the liquid phase. Now heat is transferred to the refrigerant at constant pressure until the cylinder contains vapor only. Show the process on a P-v diagram with respect to saturation lines. Determine (a) the volume occupied by the refrigerant initially, (b) the work done, and (c) the total heat transfer. Get 4.119 exercise solution
4–120 A piston–cylinder device contains helium gas initially at 150 kPa, 20°C, and 0.5 m3. The helium is now compressed in a polytropic process (PVn = constant) to 400 kPa and 140°C. Determine the heat loss or gain during this process. Get 4.120 exercise solution
4–121 A frictionless piston–cylinder device and a rigid tank initially contain 12 kg of an ideal gas each at the same temperature, pressure, and volume. It is desired to raise the temperatures of both systems by 15°C. Determine the amount of extra heat that must be supplied to the gas in the cylinder which is maintained at constant pressure to achieve this result. Assume the molar mass of the gas is 25. Get 4.121 exercise solution
4–122 A passive solar house that is losing heat to the outdoors at an average rate of 50,000 kJ/h is maintained at 22°C at all times during a winter night for 10 h. The house is to be heated by 50 glass containers each containing 20 L of water that is heated to 80°C during the day by absorbing solar energy. A thermostat-controlled 15-kW back-up electric resistance heater turns on whenever necessary to keep the house at 22°C. (a) How long did the electric heating system run that night? (b) How long would the electric heater run that night if the house incorporated no solar heating? Get 4.122 exercise solution
4–123 An 1800-W electric resistance heating element is immersed in 40 kg of water initially at 20°C. Determine how long it will take for this heater to raise the water temperature to 80°C Get 4.123 exercise solution
4–124 One ton (1000 kg) of liquid water at 80°C is brought into a well-insulated and well-sealed 4-m x 5-m x 6-m room initially at 22°C and 100 kPa. Assuming constant specific heats for both air and water at room temperature, determine the final equilibrium temperature in the room. Get 4.124 exercise solution
4–125 A 4-m x 5-m x 6-m room is to be heated by one ton (1000 kg) of liquid water contained in a tank that is placed in the room. The room is losing heat to the outside at an average rate of 8000 kJ/h. The room is initially at 20°C and 100 kPa and is maintained at an average temperature of 20°C at all times. If the hot water is to meet the heating requirements of this room for a 24-h period, determine the minimum temperature of the water when it is first brought into the room. Assume constant specific heats for both air and water at room temperature. Get 4.125 exercise solution
4–126 The energy content of a certain food is to be determined in a bomb calorimeter that contains 3 kg of water by burning a 2-g sample of it in the presence of 100 g of air in the reaction chamber. If the water temperature rises by 3.2°C when equilibrium is established, determine the energy content of the food, in kJ/kg, by neglecting the thermal energy stored in the reaction chamber and the energy supplied by the mixer. What is a rough estimate of the error involved in neglecting the thermal energy stored in the reaction chamber? Get 4.126 exercise solution
4–127 A 68-kg man whose average body temperature is 39°C drinks 1 L of cold water at 3°C in an effort to cool down. Taking the average specific heat of the human body to be 3.6 kJ/kg · °C, determine the drop in the average body temperature of this person under the influence of this cold water. Get 4.127 exercise solution
4–128 A 0.2-L glass of water at 20°C is to be cooled with ice to 5°C. Determine how much ice needs to be added to the water, in grams, if the ice is at (a) 0°C and (b) -8°C. Also determine how much water would be needed if the cooling is to be done with cold water at 0°C. The melting temperature and the heat of fusion of ice at atmospheric pressure are 0°C and 333.7 kJ/kg, respectively, and the density of water is 1 kg/L. Get 4.128 exercise solution
4–129 Reconsider Prob. 4–128. Using EES (or other) software, investigate the effect of the initial temperature of the ice on the final mass required. Let the ice temperature vary from –20 to 0°C. Plot the mass of ice against the initial temperature of ice, and discuss the results. Get 4.129 exercise solution
4–130 In order to cool 1 ton of water at 20°C in an insulated tank, a person pours 80 kg of ice at -5°C into the water. Determine the final equilibrium temperature in the tank. The melting temperature and the heat of fusion of ice at atmospheric pressure are 0°C and 333.7 kJ/kg, respectively. Get 4.130 exercise solution
4–131 An insulated piston–cylinder device initially contains 0.01 m3 of saturated liquid–vapor mixture with a quality of 0.2 at 120°C. Now some ice at 0°C is added to the cylinder. If the cylinder contains saturated liquid at 120°C when thermal equilibrium is established, determine the amount of ice added. The melting temperature and the heat of fusion of ice at atmospheric pressure are 0°C and 333.7 kJ/kg, respectively. Get 4.131 exercise solution
4–132 The early steam engines were driven by the atmospheric pressure acting on the piston fitted into a cylinder filled with saturated steam. A vacuum was created in the cylinder by cooling the cylinder externally with cold water, and thus condensing the steam. Consider a piston–cylinder device with a piston surface area of 0.1 m2 initially filled with 0.05 m3 of saturated water vapor at the atmospheric pressure of 100 kPa. Now cold water is poured outside the cylinder, and the steam inside starts condensing as a result of heat transfer to the cooling water outside. If the piston is stuck at its initial position, determine the friction force acting on the piston and the amount of heat transfer when the temperature inside the cylinder drops to 30°C. Get 4.132 exercise solution
4–133 Water is boiled at sea level in a coffee maker equipped with an immersion-type electric heating element. The coffee maker contains 1 L of water when full. Once boiling starts, it is observed that half of the water in the coffee maker evaporates in 25 min. Determine the power rating of the electric heating element immersed in water. Also, determine how long it will take for this heater to raise the temperature of 1 L of cold water from 18°C to the boiling temperature. Get 4.133 exercise solution
4–134 Two rigid tanks are connected by a valve. Tank A contains 0.2 m3 of water at 400 kPa and 80 percent quality. Tank B contains 0.5 m3 of water at 200 kPa and 250°C. The valve is now opened, and the two tanks eventually come to the same state. Determine the pressure and the amount of heat transfer when the system reaches thermal equilibrium with the surroundings at 25°C. Get 4.134 exercise solution
4–135 Reconsider Prob. 4–134. Using EES (or other) software, investigate the effect of the environment temperature on the final pressure and the heat transfer. Let the environment temperature vary from 0 to 50°C. Plot the final results against the environment temperature, and discuss the results. Get 4.135 exercise solution
4–136 A rigid tank containing 0.4 m3 of air at 400 kPa and 30°C is connected by a valve to a piston–cylinder device with zero clearance. The mass of the piston is such that a pressure of 200 kPa is required to raise the piston. The valve is now opened slightly, and air is allowed to flow into the cylinder until the pressure in the tank drops to 200 kPa. During this process, heat is exchanged with the surroundings such that the entire air remains at 30°C at all times. Determine the heat transfer for this process. Get 4.136 exercise solution
4–137 A well-insulated 4-m x 4-m x 5-m room initially at 10°C is heated by the radiator of a steam heating system. The radiator has a volume of 15 L and is filled with superheated vapor at 200 kPa and 200°C. At this moment both the inlet and the exit valves to the radiator are closed. A 120-W fan is used to distribute the air in the room. The pressure of the steam is observed to drop to 100 kPa after 30 min as a result of heat transfer to the room. Assuming constant specific heats for air at room temperature, determine the average temperature of air in 30 min. Assume the air pressure in the room remains constant at 100 kPa.

Get 4.137 exercise solution
4–138 Consider a well-insulated horizontal rigid cylinder that is divided into two compartments by a piston that is free to move but does not allow either gas to leak into the other side. Initially, one side of the piston contains 1 m3 of N2 gas at 500 kPa and 80°C while the other side contains 1 m3 of He gas at 500 kPa and 25°C. Now thermal equilibrium is established in the cylinder as a result of heat transfer through the piston. Using constant specific heats at room temperature, determine the final equilibrium temperature in the cylinder. What would your answer be if the piston were not free to move? Get 4.138 exercise solution
4–139 Repeat Prob. 4–138 by assuming the piston is made of 5 kg of copper initially at the average temperature of the two gases on both sides. Get 4.139 exercise solution
4–140 Reconsider Prob. 4–139. Using EES (or other) software, investigate the effect of the mass of the copper piston on the final equilibrium temperature. Let the mass of piston vary from 1 to 10 kg. Plot the final temperature against the mass of piston, and discuss the results. Get 4.140 exercise solution
4–141 An insulated rigid tank initially contains 1.4-kg saturated liquid water and water vapor at 200°C. At this state, 25 percent of the volume is occupied by liquid water and the rest by vapor. Now an electric resistor placed in the tank is turned on, and the tank is observed to contain saturated water vapor after 20 min. Determine (a) the volume of the tank, (b) the final temperature, and (c) the electric power rating of the resistor. Get 4.141 exercise solution
4–142 A vertical 12-cm diameter piston–cylinder device contains an ideal gas at the ambient conditons of 1 bar and 24°C. Initially, the inner face of the piston is 20 cm from the base of the cylinder. Now an external shaft connected to the piston exerts a force corresponding to a boundary work input of 0.1 kJ. The temperature of the gas remains constant during the process. Determine (a) the amount of heat transfer, (b) the final pressure in the cylinder, and (c) the distance that the piston is displaced. Get 4.142 exercise solution
4–143 A piston–cylinder device initially contains 0.15-kg steam at 3.5 MPa, superheated by 5°C. Now the steam loses heat to the surroundings and the piston moves down, hitting a set of stops at which point the cylinder contains saturated liquid water. The cooling continues until the cylinder contains water at 200°C. Determine (a) the final pressure and the quality (if mix Water ture), (b) the boundary work, (c) the amount of heat transfer when the piston first hits the stops, (d) and the total heat transfer. Get 4.143 exercise solution
4–144 An insulated rigid tank is divided into two compartments of different volumes. Initially, each compartment contains the same ideal gas at identical pressure but at different temperatures and masses. The wall separating the two compartments is removed and the two gases are allowed to mix. Assuming constant specific heats, find the simplest expression for the mixture temperature written in the form

where m3 and T3 are the mass and temperature of the final mixture, respectively. Get 4.144 exercise solution
4–145 Catastrophic explosions of steam boilers in the 1800s and early 1900s resulted in hundreds of deaths, which prompted the development of the ASME Boiler and Pressure Vessel Code in 1915. Considering that the pressurized fluid in a vessel eventually reaches equilibrium with its surroundings shortly after the explosion, the work that a pressurized fluid would do if allowed to expand adiabatically to the state of the surroundings can be viewed as the explosive energy of the pressurized fluid. Because of the very short time period of the explosion and the apparent stability afterward, the explosion process can be considered to be adiabatic with no changes in kinetic and potential energies. The closed-system conservation of energy relation in this case reduces to Wout = m(u1 – u2).

Then the explosive energy Eexp becomes Eexp = m(u1 - u2) where the subscripts 1 and 2 refer to the state of the fluid before and after the explosion, respectively. The specific where the subscripts 1 and 2 refer to the state of the fluid before and after the explosion, respectively. The specific where v1 is the specific volume of the fluid before the explosion.

Show that the specific explosion energy of an ideal gas with constant specific heat is Also, determine the total explosion energy of 20 m3 of air at 5 MPa and 100°C when the surroundings are at 20°C. Get 4.145 exercise solution
4–146 Using the relations in Prob. 4–145, determine the explosive energy of 20 m3 of steam at 10 MPa and 500°C assuming the steam condenses and becomes a liquid at 25°C after the explosion. To how many kilograms of TNT is this explosive energy equivalent? The explosive energy of TNT is about 3250 kJ/kg. Get 4.146 exercise solution
4–147 A room is filled with saturated steam at 100°C. Now a 5-kg bowling ball at 25°C is brought to the room. Heat is transferred to the ball from the steam, and the temperature of the ball rises to 100°C while some steam condenses on the ball as it loses heat (but it still remains at 100°C). The specific heat of the ball can be taken to be 1.8 kJ/kg · C. The mass of steam that condensed during this process is (a) 80 g (b) 128 g (c) 299 g (d) 351 g (e) 405 g Get 4.147 exercise solution
4–148 A frictionless piston–cylinder device and a rigid tank contain 2 kmol of an ideal gas at the same temperature, pressure, and volume. Now heat is transferred, and the temperature of both systems is raised by 10°C. The amount of extra heat that must be supplied to the gas in the cylinder that is maintained at constant pressure is (a) 0 kJ (d) 102 kJ (b) 42 kJ (e) 166 kJ (c) 83 kJ Get 4.148 exercise solution
4–149 The specific heat of a material is given in a strange unit to be c 3.60 kJ/kg °F. The specific heat of this material in the SI units of kJ/kg °C is (a) 2.00 kJ/kg · °C (d) 4.80 kJ/kg · °C (b) 3.20 kJ/kg · °C (e) 6.48 kJ/kg · °C (c) 3.60 kJ/kg · °C Get 4.149 exercise solution
4–150 A 3-m3 rigid tank contains nitrogen gas at 500 kPa and 300 K. Now heat is transferred to the nitrogen in the tank and the pressure of nitrogen rises to 800 kPa. The work done during this process is (a) 500 kJ (d) 900 kJ (b) 1500 kJ (e) 2400 kJ (c) 0 kJ Get 4.150 exercise solution
4–151 A 0.8-m3 rigid tank contains nitrogen gas at 600 kPa and 300 K. Now the gas is compressed isothermally to a volume of 0.1 m3. The work done on the gas during this compression process is (a) 746 kJ (d) 998 kJ (b) 0 kJ (e) 1890 kJ (c) 420 kJ Get 4.151 exercise solution
4–152 A well-sealed room contains 60 kg of air at 200 kPa and 25°C. Now solar energy enters the room at an average rate of 0.8 kJ/s while a 120-W fan is turned on to circulate the air in the room. If heat transfer through the walls is negligible, the air temperature in the room in 30 min will be (a) 25.6°C (d) 52.5°C (b) 49.8°C (e) 63.4°C (c) 53.4°C Get 4.152 exercise solution
4–153 A 2-kW baseboard electric resistance heater in a vacant room is turned on and kept on for 15 min. The mass of the air in the room is 75 kg, and the room is tightly sealed so that no air can leak in or out. The temperature rise of air at the end of 15 min is (a) 8.5°C (d) 33.4°C (b) 12.4°C (e) 54.8°C (c) 24.0°C Get 4.153 exercise solution
4–154 A room contains 60 kg of air at 100 kPa and 15°C. The room has a 250-W refrigerator (the refrigerator consumes 250 W of electricity when running), a 120-W TV, a 1kW electric resistance heater, and a 50-W fan. During a cold winter day, it is observed that the refrigerator, the TV, the fan, and the electric resistance heater are running continuously but the air temperature in the room remains constant. The rate of heat loss from the room that day is (a) 3312 kJ/h (d) 2952 kJ/h (b) 4752 kJ/h (e) 4680 kJ/h (c) 5112 kJ/h Get 4.154 exercise solution
4–155 A piston–cylinder device contains 5 kg of air at 400 kPa and 30°C. During a quasi-equilibium isothermal expansion process, 15 kJ of boundary work is done by the system, and 3 kJ of paddle-wheel work is done on the system. The heat transfer during this process is (a) 12 kJ (d) 3.5 kJ (b) 18 kJ (e) 60 kJ (c) 2.4 kJ Get 4.155 exercise solution
4–156 A container equipped with a resistance heater and a mixer is initially filled with 3.6 kg of saturated water vapor at 120°C. Now the heater and the mixer are turned on; the steam is compressed, and there is heat loss to the surrounding air. At the end of the process, the temperature and pressure of steam in the container are measured to be 300°C and 0.5 MPa. The net energy transfer to the steam during this process is (a) 274 kJ (d) 988 kJ (b) 914 kJ (e) 1291 kJ (c) 1213 kJ Get 4.156 exercise solution
4–157 A 6-pack canned drink is to be cooled from 25°C to 3°C. The mass of each canned drink is 0.355 kg. The drinks can be treated as water, and the energy stored in the aluminum can itself is negligible. The amount of heat transfer from the 6 canned drinks is (a) 33 kJ (d) 196 kJ (b) 37 kJ (e) 223 kJ (c) 47 kJ Get 4.157 exercise solution
4–158 A glass of water with a mass of 0.45 kg at 20°C is to be cooled to 0°C by dropping ice cubes at 0°C into it. The latent heat of fusion of ice is 334 kJ/kg, and the specific heat of water is 4.18 kJ/kg · °C. The amount of ice that needs to be added is (a) 56 g (d) 224 g (b) 113 g (e) 450 g (c) 124 g Get 4.158 exercise solution
4–159 A 2-kW electric resistance heater submerged in 5-kg water is turned on and kept on for 10 min. During the process, 300 kJ of heat is lost from the water. The temperature rise of water is (a) 0.4°C (d) 71.8°C (b) 43.1°C (e) 180.0°C (c) 57.4°C Get 4.159 exercise solution
4–160 3 kg of liquid water initially at 12°C is to be heated at 95°C in a teapot equipped with a 1200-W electric heating element inside. The specific heat of water can be taken to be 4.18 kJ/kg · °C, and the heat loss from the water during heating can be neglected. The time it takes to heat water to the desired temperature is (a) 4.8 min (d) 9.0 min (b) 14.5 min (e) 18.6 min (c) 6.7 min Get 4.160 exercise solution
4–161 An ordinary egg with a mass of 0.1 kg and a specific heat of 3.32 kJ/kg · °C is dropped into boiling water at 95°C. If the initial temperature of the egg is 5°C, the maximum amount of heat transfer to the egg is (a) 12 kJ (d) 18 kJ (b) 30 kJ (e) infinity (c) 24 kJ Get 4.161 exercise solution
4–162 An apple with an average mass of 0.18 kg and average specific heat of 3.65 kJ/kg · °C is cooled from 22°C to 5°C. The amount of heat transferred from the apple is (a) 0.85 kJ (d) 11.2 kJ (b) 62.1 kJ (e) 7.1 kJ (c) 17.7 kJ Get 4.162 exercise solution
4–163 The specific heat at constant pressure for an ideal gas is given by cp = 0.9 x (2.7 x 10-4)T (kJ/kg · K) where T is in kelvin. The change in the enthalpyfor this ideal gas undergoing a process in which the temperature changes from 27 to 127°C is most nearly (a) 90 kJ/kg (d) 108.9 kJ/kg (b) 92.1 kJ/kg (e) 105.2 kJ/kg (c) 99.5 kJ/kg Get 4.163 exercise solution
4–164 The specific heat at constant volume for an ideal gas is given by cv = 0.7 + (2.7 x 10-4)T (kJ/kg · K) where T is in kelvin. The change in the internal energy for this ideal gas undergoing a process in which the temperature changes from 27 to 127°C is most nearly (a) 70 kJ/kg (d) 82.1 kJ/kg (b) 72.1 kJ/kg (e) 84.0 kJ/kg (c) 79.5 kJ/kg Get 4.164 exercise solution
4–165 A piston–cylinder device contains an ideal gas. The gas undergoes two successive cooling processes by rejecting heat to the surroundings. First the gas is cooled at constant pressure until T2 = 3/4T1. Then the piston is held stationary while the gas is further cooled to T3 = 1/2T1, where all temperatures are in K. 1. The ratio of the final volume to the initial volume of the gas is (a) 0.25 (d) 0.75 (b) 0.50 (e) 1.0 (c) 0.67 2. The work done on the gas by the piston is (a) RT1/4 (d) (cv + cp)T1/4 (b) cvT1/2 (e) cv (T1 + T2)/2 (c) cpT1/2 3. The total heat transferred from the gas is (a) RT1/4 (d) (cv + cp)T1/4 (b) cvT1/2 (e) cv (T1 + T3)/2 (c) cpT1/2 Get 4.165 exercise solution
4–166 Saturated steam vapor is contained in a piston–cylinder device. While heat is added to the steam, the piston is held stationary, and the pressure and temperature become 1.2 MPa and 700°C, respectively. Additional heat is added to the steam until the temperature rises to 1200°C, and the piston moves to maintain a constant pressure. 1. The initial pressure of the steam is most nearly (a) 250 kPa (d) 1000 kPa (b) 500 kPa (e) 1250 kPa (c) 750 kPa 2. The work done by the steam on the piston is most nearly (a) 230 kJ/kg (d) 2340 kJ/kg (b) 1100 kJ/kg (e) 840 kJ/kg (c) 2140 kJ/kg 3. The total heat transferred to the steam is most nearly (a) 230 kJ/kg (d) 2340 kJ/kg (b) 1100 kJ/kg (e) 840 kJ/kg (c) 2140 kJ/kg Get 4.166 exercise solution
4–172 It is claimed that fruits and vegetables are cooled by 6°C for each percentage point of weight loss as moisture during vacuum cooling. Using calculations, demonstrate if this claim is reasonable. Get 4.172 exercise solution